the butterfly effect
In 1963, Edward Lorenz (1917-2008), studied convection in the Earth's atmosphere. As the Navier-Stokes equations that describe fluid dynamics are very difficult to solve, he simplified them drastically. The model he obtained probably has little to do with what really happens in the atmosphere. It is a toy-model, but Lorenz soon realised that it as very interesting in a mathematical sense. There are only three parameters in the model so that each point (x,y,z) symbolises a state of the atmosphere.
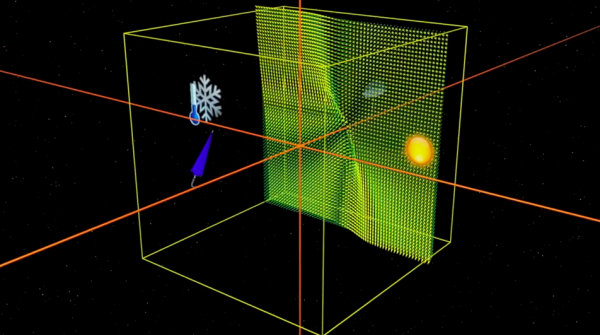

The evolution of the weather thus boils down to following trajectories in a vector field. Again, it is a toy model, and the objective is to try and understand some very complex behaviour.

Two conditions of the atmosphere that are extremely close will rapidly evolve quite differently: after a while they represent conditions that are wide apart. Lorenz discovered this sensitivity to initial conditions in his model.
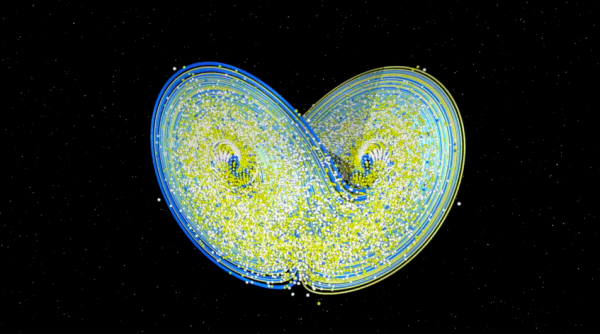
If we take a large number of different initial conditions, then after a while they all land on the same object in the shape of a butterfly: the Lorenz attractor. A strange attractor!
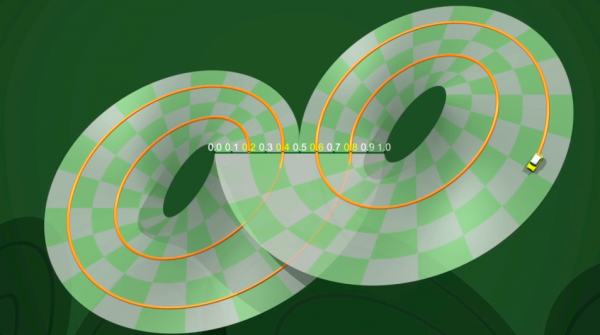
Understanding the Lorenz attractor is quite a task! How do the internal dynamics behave? Birman, Guckenheimer et Williams proposed a model in the 1970's that one can construct with nothing more than a strip of paper. With this simple model we can look at the dynamics in discrete time.
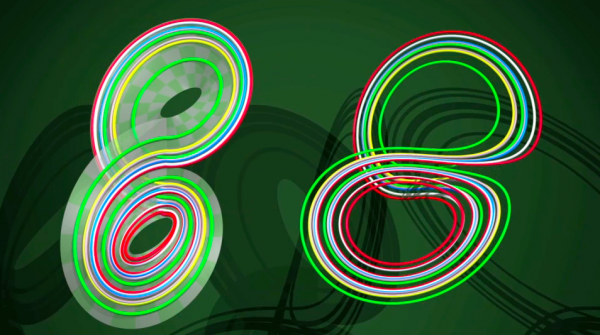
Only in 2001 did mathematician Warwick Tucker prove that the paper model accurately describes the motion on the Lorenz attractor. For every trajectory on the attractor, there is a trajectory on the paper model that behaves exactly the same way.
All this is of course very simplistic in comparison with the real weather phenomena, but it illustrates the fact that mathematicians love simple things!
